Work done by Force
Work done by a force acting on an object.
When a body is moved as a result of a force being applied to it - work is done .
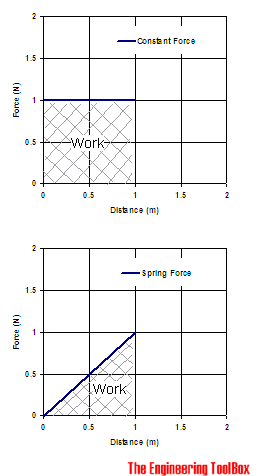
Work done by a Constant Force
The amount of work done by a constant force can be expressed as
WF = F s (1)
where
WF = work done (J, ft lbf)
F = constant force acting on object (N, lbf)
s = distance object is moved in direction of force (m, ft)
The unit of work in SI units is joule (J) which is defined as the amount of work done when a force of 1 Newton acts for distance of 1 m in the direction of the force.
- 1 J (Joule) = 0.1020 kpm = 2.778×10-7 kWh = 2.389×10-4 kcal = 0.7376 ft lbf = 1 (kg m2)/s2= 1 watt second = 1 Nm = 1 ft lb = 9.478×10-4 Btu
- 1 ft lbf (foot pound force) = 1.3558 J = 0.1383 kp m = 3.766×10-7 kWh = 3.238×10-4 kcal = 1.285×10-3 Btu
This is the same unit as energy.
The work done by a constant force is visualized in the chart above. The work is the product force x distance and represented by the area as indicated in the chart.
Example - Constant Force and Work
A constant force of 20 N is acting a distance of 30 m. The work done can be calculated as
WF = (20 N) (30 m)
= 600 (J, Nm)
Example - Work done when lifting a Brick of mass 2 kg a height of 20 m above ground
The force acting on the brick is the weight and the work can be calculated as
WF = F s
= m ag s (2)
= (2 kg) (9.81 m/s2) (20 m)
= 392 (J, Nm)
Example - Work when Climbing Stair - Imperial units
The work made by a person of 150 lb climbing a stair of 100 ft can be calculated as
WF = (150 lb) (100 ft)
= 15000 ft lb
Work done by a Spring Force
The force exerted by springs varies with the extension or compression of the spring and can be expressed with Hooke's Law as
Fspring = - k s (3)
where
Fspring = spring force (N, lbf)
k = spring constant
The work done by a spring force is visualized in the chart above. The force is zero with no extension or compression and the work is the half the product force x distance and represented by the area as indicated. The work done when a spring is compressed or stretched can be expressed as
Wspring = 1/2 Fspring_max s
= 1/2 k s2 (4)
where
Wspring = work done (J, ft lbf)
Fspring_max = maximum spring force (N, lbf)
Example - Spring Force and Work
A spring is extended 1 m. The spring force is variable - from 0 N to 1 N as indicated in the figure above - and the work done can be calculated as
Wspring = 1/2 (1 N/m) (1 m)2
= 0.5 (J, Nm)
The spring constant can be calculated by modifying eq. 4 to
k = 2 (0.5 J)/ (1 m)2
= 1 N/m
Work done by Moment and Rotational Displacement
Rotational work can be calculated as
WM = T θ (5)
where
WM = rotational work done (J, ft lb)
T = torque or moment (Nm, ft lb)
θ = displacement angle (radians)
Example - Rotational Work
A machine shaft acts with moment 300 Nm . The work done per revolution (2 π radians) can be calculated as
WM = (300 Nm) (2 π)
= 1884 J
Representations of Work
Force can be exerted by weight or pressure:
W = ∫ F ds
= ∫ m ag dh
=∫ p A ds
=∫ p dV (6)
where
W = work (J, Nm)
F = force (N)
ds = distance moved for acting force, or acting pressure (m)
m = mass (kg)
ag = acceleration of gravity (m/s2)
dh = elevation for acting gravity (m)
p = pressure on a surface A, or in a volume (Pa, N/m2)
A = surface for acting pressure (m2)
dV = change in volume for acting pressure p (m3)
Power vs. Work
Power is the ratio of work done to used time - or work done per unit time.



